Calibration in regression.

lmCalib computes the estimate of \(x_0\) in which the regression of \(y ~ x\) reaches given \(y0\), and the related confidence interval.

lmCalib(x, y, y0, fixed = FALSE, approx = FALSE, alpha = 0.05)

Arguments

x

independent variable
y

dependent variable
y0

dependent variable for which to compute the expected independent variable
fixed

is y0 fixed, or random?
approx

use approximation, or Fieller method?
alpha

confidence interval significance level

Details

The code is taken from Appendix A.4.5 in Zvara2008. The estimation is based on chapter 4.4. in Zvara2008

Value

A vector of the estimate of 'x0', and lower and upper limits of related confidence interval.

References

Karel Zv\'ara: Regrese, Matfyzpress Praha 2008, J.A. Neter, W. Wasserman, M. Kutner: Applied Linear Statistical Models, Irwin, Homewood, Illinois (1985).

See also

lmInt

Examples

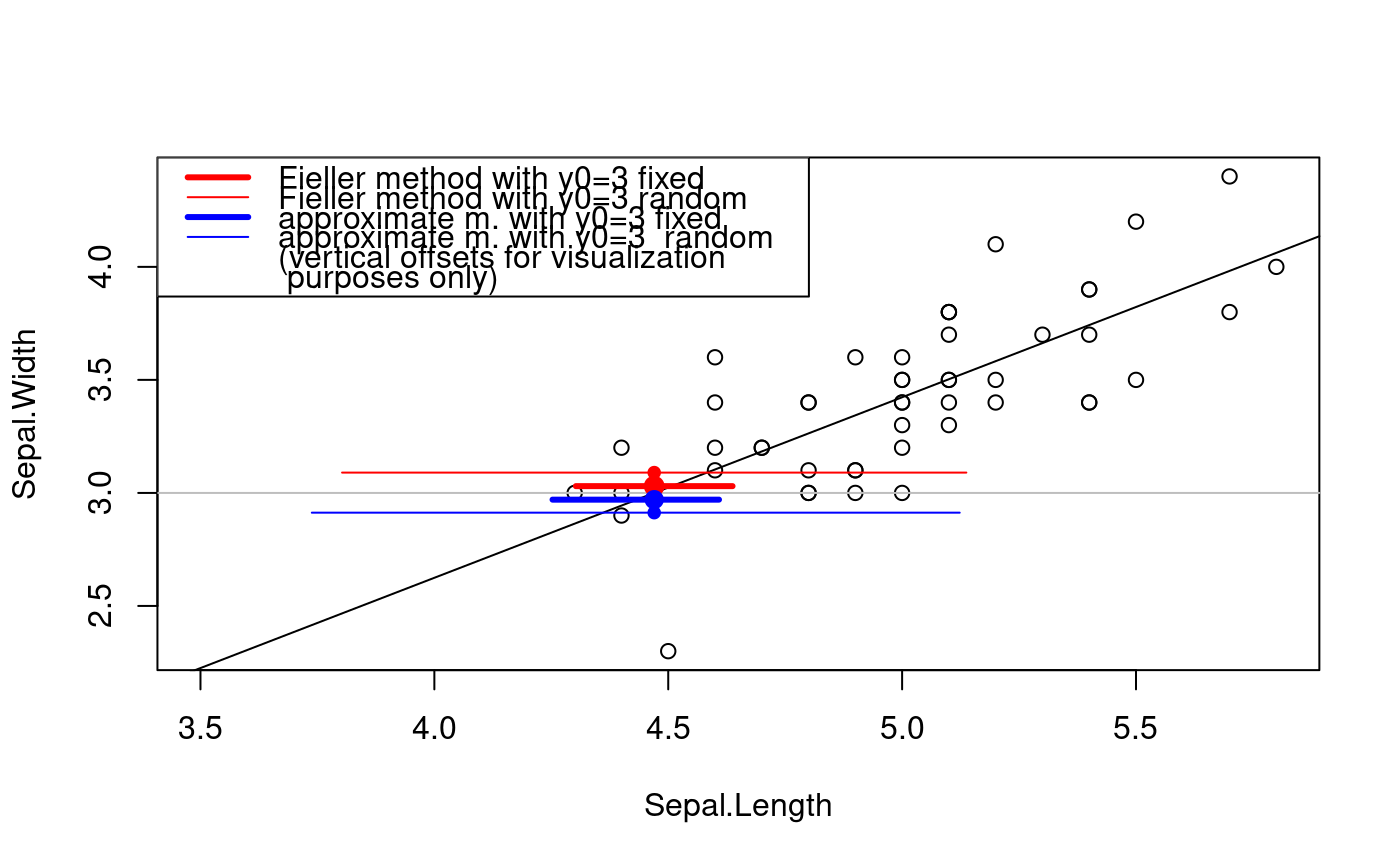
iris.setosa<-iris[iris$Species=='setosa',]
attach(iris.setosa)

plot(Sepal.Length, Sepal.Width, xlim=c(min(3.5,min(Sepal.Length)),max(Sepal.Length)))
abline(coef(lm(Sepal.Width ~ Sepal.Length)))

y0 <- 3
abline(h = y0, col='gray')

# Fieller method with y0 fixed
calib <- lmCalib(Sepal.Length, Sepal.Width, y0, fixed = TRUE, approx = TRUE)
lines(calib[2:3],rep(y0,2)*1.01,col='red',lwd=3)
points(calib[1],y0*1.01,pch=19,cex=1.3,col='red')
# Fieller method with y0 random
calib <- lmCalib(Sepal.Length, Sepal.Width, y0, fixed = FALSE, approx = TRUE)
lines(calib[2:3],rep(y0,2)*1.03,col='red',lwd=1)
points(calib[1],y0*1.03,pch=19,cex=.8,col='red')

# approximate method with y0 fixed
calib <- lmCalib(Sepal.Length, Sepal.Width, y0, fixed = TRUE, approx = FALSE)
lines(calib[2:3],rep(y0,2)/1.01,lwd=3,col='blue')
points(calib[1],y0/1.01,pch=19,cex=1.2,col='blue')
# approximate method with y0 random
calib <- lmCalib(Sepal.Length, Sepal.Width, y0, fixed = FALSE, approx = FALSE)
lines(calib[2:3],rep(y0,2)/1.03,lwd=1,col='blue')
points(calib[1],y0/1.03,pch=19,cex=.8,col='blue')

legend('topleft', bg='white',
  c(paste0('Fieller method with y0=',y0,' fixed'),
    paste0('Fieller method with y0=',y0,' random'),
    paste0('approximate m. with y0=',y0,' fixed'),
    paste0('approximate m. with y0=',y0,'  random'),
    '(vertical offsets for visualization',
    ' purposes only)'),
  col = c('red', 'red', 'blue', 'blue', 'black'),
  lwd = c(3, 1, 3, 1, NA, NA))

detach(iris.setosa)